Some of you know that we have a Sommerer Family Website. Recently I posted some pages from a notebook that my Grandpa used from 1937 to about 1945. It’s where he kept notes about farm stuff. I find it fascinating in general, but I was especially intrigued by what appeared to be random ciphering on some of the pages…
First, a quick summary:
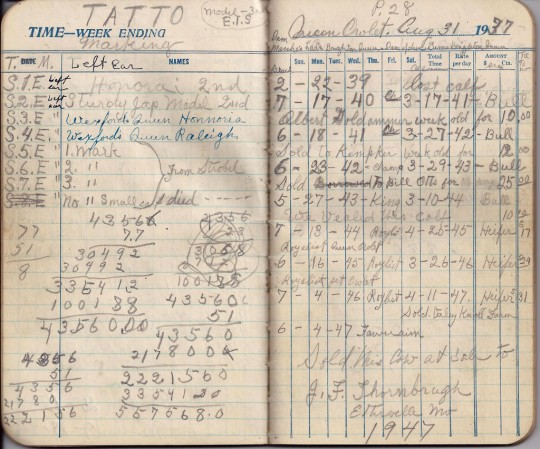
- 77 + 51 = (not finished)
- 4356 x 23 = 100188 (scribbled out)
- 4356 x 7.7 = 33541,2 + 100188 = 43560.00
- 4356 x 51 = 222156
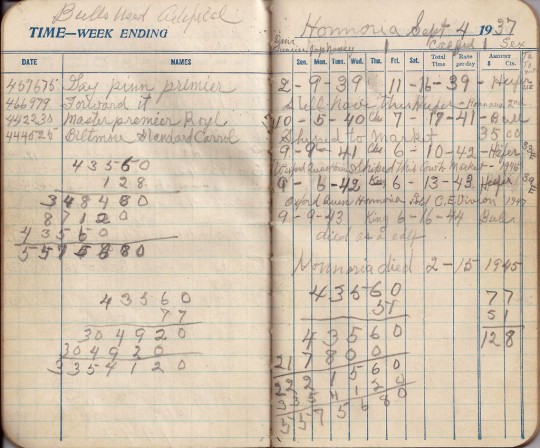
- 43560 x 51 = 2221560 + 3357120 = 5575680
- 43560 x 51 = 2221560
- 43560 x 77 = 3354120
- 43560 x 51 = 2221560 + 3354120 = 5575680
- 77 + 51 = 128
- 43560 x 128 = 5575680
So, we see from 5, 8 & 10 that figuring out the correct answer to 128 x 43560 is important enough that Grandpa did it 3 times in 3 different ways. But why would that be so important? And although that’s what the over half of the ciphering is about, what is the importance of 2, 3 & 4 which appear only somewhat related.
I spent a fair amount of time looking at the numbers 77, 51, 23, 128 and 4356 thinking that that was the key to the problem. In hind-sight that was obviously the wrong take. I was thrown off by the 4356 and didn’t really notice the 43560.
Twenty years ago I was really familiar with the number 43560. Twenty years ago a routine part of my summer was figuring out what the average number of corn plants was per acre in a corn field. 43560 is the number of square feet in an acre.
So it seems pretty obvious now that Grandpa had a good reason to figure out that square feet in a field of 128 acres or maybe the total square feet in fields of 77 and 51 acres.
But those are really big fields for Mid Missouri in the 30s and 40s. So maybe they were fields of 7.7 and 5.1 acres respectively? Cipher #3 seems to point in that direction. Maybe Grandpa just figured he would drop the decimal point and just lop-off a zero at the end (was grandpa good at math?).
So then the only odd calculation is #2. Where does that 23 come in? Is there a field of 23 acres (or 2.3 acres) somewhere? Why doesn’t it enter into the calculations at the end like the others do.
But then a really odd number jumped out at me. The calculation for #3 uses the answer to #2. And the answer to #3 is our old friend 43560, the number of square feet in an acre. Did Grandpa just derive the number of square feet in an acre? It seems like a really strange coincidence for that particular number to come up in this context otherwise.
And if that’s what he did, how did he do it? I can never remember the number of square feet in an acre either, but I do remember that there are 5280 feet in a mile and that a square mile is 640 acres, so it’s easy to get square feet in an acre from that. But what about those other numbers? How does (4356 x 23) + (4356 x 7.7) give you the number of square feet in an acre?
And, of course, why did Grandpa need to know the number of square feet in those particular fields?
